Physical Chemistry Practical (MS)

 Physical Chemistry Practical (MS)

TITLE:

Determination of Phase Diagram for Ethanol/Toluene/Water System Theory

OBJECTIVES:

1. To determine the solubility limits in a ternary system of water and two other liquids  (ethanol and toluene), where one of which is completely miscible (ethanol) and the other is partly miscible with water (toluene).

2. To apply the concept of construction of the solubility curve of the system being studied on triangular diagram.

3. To understand the concept of miscibility and phase diagram for three-component system.

4. To understand Phase Rules that relate to the use of triangular coordinates to know the mutual solubility of liquids in a two phase system.

INTRODUCTION    

             Following the basis of describing the effect of intensive variable to various phase in a system at equilibrium, which is the phase rule, it is determine that this system have 4 degrees of freedom. The four degrees of freedom are - temperature, pressure, and any two from the three component concentration. 

F = C – P + 2                          where F refers to Degrees of Freedom;

F = 3 – 1 + 2                                      C refers to Component concentration;

F = 4                                                   P refers to Phase.

             In this experiment, there are three components of concern which were Ethanol, Toluene and Water. Water is insoluble and toluene, but as it was mixed together with ethanol, all three components can achieve homogeneous solution at equilibrium if proper proportion was used. Since it is difficult to graphically represent four variables, one variable out of the four is generally considered constant. In this experiment, the pressure is considered fixed at 1 atm, and so the number of degrees of freedom becomes three. Any horizontal section of the right-angled prism represents a three-component system under fixed condition of temperature and pressure. 

            For three component systems at constant temperature and pressure, the compositions may be stated in the form of coordinates for a triangular diagram.

Click for Video lesson

https://www.youtube.com/watch?app=desktop&v=LTHR92z2_Y0

Figure 1: Ternary Phase Diagram

          In the diagram above, each corner of the triangular diagram represents a pure component, which is 100% A, 100% B, 100% C. Meanwhile, each side represents two-component mixtures and within the triangular diagram itself represents ternary components. Any line parallel to a side of the triangular diagram shows constant percentage value for a component, for example: DE shows 20% of A with varying amounts of B and C. So does line FG, showing all mixtures containing 50% of B. These lines intercept with each other at K, which definitely contains 20% A, 50% B as well as 30% C. Measurements can be made this way because in a triangular diagram, the sum of all distances from K which is drawn parallel to the three sides of the diagram is same and equals to the length of any one side of the triangular diagram.

          The addition of a third component to a pair of miscible liquids can change their mutual solubility. If this third component is more soluble in one of two different components the mutual solubility of the liquid pair is decreased. However, if it is soluble in both of the liquids, the mutual solubility is increased. Thus, when ethanol is added to a mixture of benzene and water, the mutual solubility of the liquid pair increased until it reached a point whereby the mixture becomes homogenous. This approach is used in the formulation of solutions. Examples of three-component systems that has been studied include castor oil/ alcohol/ water; peppermint oil/ propylene glycerol/ water ; peppermint oil/ polyethylene glycerol/ water.

 

PROCEDURE

1) 20 mL of a mixture of ethanol and toluene with 10% ethanol was prepared by transferring 2 mL of ethanol and 18 mL toluene with a 20 mL pipette into a conical flask.
2) A 50 mL burette was filled with water
3) The mixture was immediately titrated with water until it becomes cloudy.
4) The mixture was then added with a little water by using dropper and the conical flask was shaken well.
5) The volume of water used in titration was recorded.
6) Another 20 mL of mixture of ethanol and toluene was prepared for second titration in order to obtain an average volume of water used.
7) Step 1 to 5 were repeated by using mixture of ethanol and toluene with various ethanol percentages which consists of 25%, 35%, 50%, 65%, 75%, 90%, and 95%.
8) The room temperature was measured.
9) The percentage of each component in the mixture after addition of water is calculated.
10) The points were plotted onto a triangular paper to give a triple phase diagram at the recorded temperature.

------------------------------------------------------------------------

Lab work 1:   Determination of the critical micelle concentration of a surfactant

• Objective

The main objective of this lab work is to determine the critical micelle concentration of an anionic surfactant, sodium dodecyl sulfate (SDS), with two different techniques: a) surface tension measurements using the Du Nouy ring method; and b) conductivity measurements. The effect of salt on the critical micelle concentration will be also analyzed in the latter case.

• Introduction

Surfactants are water-soluble amphiphilic molecules that consist of a non-polar hydrophobic part (usually a hydrocarbon or fluorocarbon chain) and a polar hydrophilic part (head group). The hydrophilic head group can be nonionic, anionic, cationic, or zwitterionic. The balance between hydrophobic and hydrophilic parts gives special properties to surfactants, e.g. high affinity to adsorb at interfaces and association in solution to form micelles.

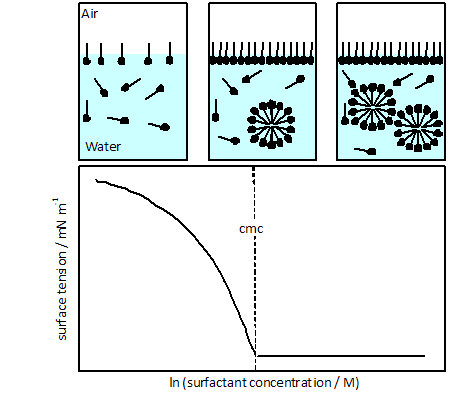
The concentration at which surfactants start to form micelles is called critical micelle concentration (c.m.c.). Each surfactant has a characteristic c.m.c. at a given temperature and salt concentration. The c.m.c. of a surfactant can be obtained by different techniques, which in general are based on the measurement of a magnitude that shows an abrupt change at c.m.c. The surface tension at the air/water interface and the electrical conductivity of a solution are examples of such magnitudes.

The surface tension at the air/water interface decreases when the surfactant concentration in the aqueous solution increases. This effect is due to the adsorption of surfactant molecules at the interface. The drop of surface tension stops when the surfactant starts to form micelles in solution (c.m.c.). The surface tension remains virtually constant at surfactant concentrations above the c.m.c. Therefore, the c.m.c. can be determined from surface tension measurements at different surfactant concentrations. The inflection point in graphs of surface tension versus logarithm of surfactant concentration gives the c.m.c. There are different methods to measure the surface tension of an air/liquid interface. In this lab work the surface tension will be measured using the Du Nouy ring method (see Appendix).

The electrical conductivity is a magnitude that describes the ability of a material or solution to conduct an electric current. The electrical conductivity of a solution depends on the number and mobility of ions and charged particles present in the solution. In the case of ionic surfactants, the electrical conductivity increases as the surfactant concentration increases. The formation of micelles affect the conductivity of the solution. The c.m.c. can be obtained from the inflection point (intersection of linear fits) in the curve conductivity versus surfactant concentration. 

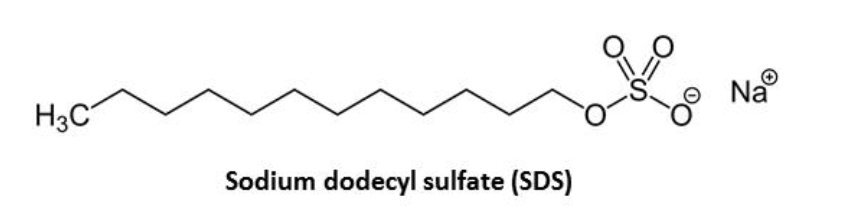
Sodium dodecyl sulfate (SDS), also called sodium lauryl sulfate, is an anionic surfactant commonly used in many cleaning and hygiene products. Its c.m.c. will be determined in this lab work with two different techniques:

a) Surface tension measurements using the Du Nouy ring method.

b) Conductivity measurements.

Lab work 1.a)  Determination of c.m.c. of SDS by surface tension measurements.

The surface tension of different SDS solutions is measured with a KSV Sigma70 tensiometer or a Krüss tensiometer using the Du Nouy ring method. Please carry out the lab work according to the following instructions:

• Clean properly the vessel and the Du Nouy ring with aqua regia (HNO3 : HCl, 1:3) and deionized water. Burn the ring in the flame of a Bunsen burner to remove any impurity.

• Add 30 ml of deionized water to the vessel and place it on the stage of the tensiometer. Hang the clean ring on the balance hook over the vessel.

• If you are using the KSV Sigma70 tensiometer, open the software sgserver and click on the Surface Tension Measurement icon. Click File 🡪 New Experiment. Choose a name for the experiment. Probe: standard ring. Vessel: small. Heavy phase: water (volume = 30 ml). Light phase: air. Press the Start button and check the parameters in the next window: Speed up = 5 mm/min; Speed down = 20 mm/min; Dwell down = 5%; Minimum number of points = 10; Minimum measurement time = 0 s; Wait before start = 0 s; AutoZero: enabled. Press the Start button to measure the surface tension of deionized water.

• If you are using the Krüss tensiometer, set the instrument at 0 mN/m when the ring is hung in the air. Lift the vessel stage with the screw and immerse the ring in the water. Turn the scale knob and write down the value of surface tension just before the ring is pulled out the water.

• Prepare 10 ml of two solutions of SDS at 120 mM and 320 mM (MWSDS = 288.37 g/mol). Repeat the process of surface tension measurement after adding different volumes of the SDS solutions to the water in the vessel according to the following table:

Volume of SDS solution added to the vessel	Total volume  in the vessel (ml)	SDS concentration  in the vessel (mM)	Surface tension (mN/m)
0	30	0
100 μl of 120 mM SDS
Plus 50 μl of 120 mM SDS
Plus 100 μl of 120 mM SDS
Plus 150 μl of 120 mM SDS
Plus 120 μl of 120 mM SDS
Plus 150 μl of 120 mM SDS
Plus 130 μl of 120 mM SDS
Plus 220 μl of 120 mM SDS
Plus 280 μl of 120 mM SDS
Plus 340 μl of 120 mM SDS
Plus 510 μl of 120 mM SDS
Plus 550 μl of 120 mM SDS
Plus 1 ml of 120 mM SDS
Plus 740 μl of 320 mM SDS
Plus 1.3 ml of 320 mM SDS
Plus 2.5 ml of 320 mM SDS

• Clean everything after finishing the experiment.

Lab work 1.b)  Determination of c.m.c. of SDS by conductivity measurements.

A conductimeter is used to measure the conductivity of a SDS solution. The concentration of SDS in the solution is increased by continuous addition of other concentrated SDS solution with a dispenser. The measurements are accomplished in the absence and presence of 10 mM NaCl. Please carry out the lab work according to the following instructions:

1. - Prepare 50 ml of a solution 320 mM SDS (MWSDS = 288.37 g/mol).

- Introduce the conductivity electrode in 400 ml deionized water and write the conductivity value. Gently stir the solution with a magnetic stirrer for the whole experiment.

- Add 0.5 ml of 320 mM SDS solution with the dispenser and write the new value of conductivity (wait until the value is stable). Avoid the presence of air bubbles in the dispenser tube.

- Repeat the process until the total volume of 320 mM SDS solution added is 25 ml.

2. - Clean the dispenser with deionized water.

- Prepare 50 ml of a solution 160 mM SDS, 10 mM NaCl (MWNaCl = 58.44 g/mol).

- Introduce the conductivity electrode in 400 ml of 10 mM NaCl solution and write the conductivity value. Gently stir the solution with a magnetic stirrer for the whole experiment.

- Add 0.5 ml of 160 mM SDS, 10 mM NaCl solution with the dispenser and write the new value of conductivity (wait until the value is stable). Avoid the presence of air bubbles in the dispenser tube.

- Repeat the process until the total volume of 160 mM SDS, 10 mM NaCl solution added is 25 ml. 

- Clean everything after finishing the experiment. 

• Homework

- Fill in the table for lab work 1.a) and plot the experimental data of surface tension versus logarithm of surfactant concentration. Determine the c.m.c. of SDS from the graph.

- Present in tables and plot the experimental data of conductivity versus surfactant concentration for the cases of no NaCl and 10 mM NaCl added to the system. Determine the c.m.c. of SDS from the graphs (intersection of linear fits).

- Compare the c.m.c. values obtained from surface tension measurements and conductivity measurements (no NaCl added) with the value found in the literature. Discuss the results.

- Discuss the effect of NaCl on the c.m.c. of SDS.

• Additional information

- This lab work is carried out in groups of 4 people: 2 people for lab work 1.a) and 2 people for lab work 1.b). They should share the results of the experiments, analyze the data together, and submit the answers to the homework in a common report within one week.

- Up to 3 points can be obtained for this lab work: up to 1 point for the work in the lab, and up to 2 points for the report with the answers to the homework.

- Safety in the lab is a very important issue. You must always wear lab coat, gloves and goggles when you work in the lab.

 

 

https://www.biolinscientific.com/measurements/critical-micelle-concentration