Quantum Mechanics (4H)

QUANTUM MECHANICS (3H)

Quantum Chemistry Syllabus:

1. 1. Quantum mechanics and its necessity,
2. 2. experiments supporting quantization concept of electromagnetic radiation, blackbody radiation,
3. 3. photoelectric effect,
4. 4. Compton effect,
5. 5. spectroscopic observations;
6. 6. de Broglie’s wave particle dualism; matter waves;
7. 7. double-slit experiment
8. 8. Heisenberg’s uncertainty principle.
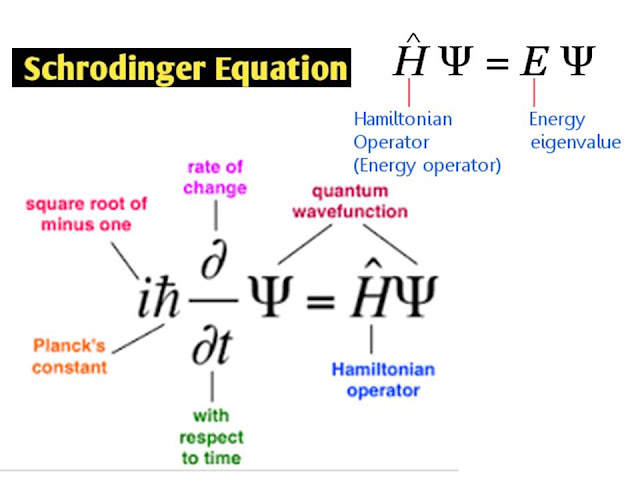
9. 9. Dynamics of microcopic systems: Schrodinger’s wave equation, its deduction,
10. 10. particle in a box problem: one and three dimensional boxes,
11. 11. Eigen functions and Eigen values,
12. 12. Schrodinger’s wave equation for H-atom,
13. 13. separation of radial and angular functions,
14. 14. solutions for wave functions,
15. 15. derivation of different quantum numbers,
16. 16. wave functions for different orbitals,
17. 17. orbital shapes and orientations for H-like atom.

1. Quantum mechanics and its necessity (Overview):

1. 2. experiments supporting quantization concept of electromagnetic radiation,
2. blackbody radiation:

1. 3. photoelectric effect:

 

 

Numerical problem on Photoelectric effect

https://thefactfactor.com/facts/pure_science/physics/threshold-frequency/4877/ 

 Compton effect:

1. 
   
2. 
3. 
   
4. λi is initial wavelength, λf  is the wavelength after scattering, m0 is the electron rest mass, c is the speed of light, and θ is the scattering angle.

• Compton scattering was discovered by Arthur Holly Compton.
• Compton scattering is the scattering of a photon by a charged particle, usually an electron. 
• It results in a decrease in energy (increase in wavelength) of the photon (which may be an X-ray or gamma ray photon), called the Compton effect. 
• Part of the energy of the photon is transferred to the recoiling electron. 
• Inverse Compton scattering occurs when a charged particle transfers part of its energy to a photon.

....

 de-Broglie equation

 

- Particles can have wave-like properties and Waves can have particle-like properties,

- The universe on the smallest scale is “quantised” – matter and energy exist in tiny “packets” 

photons of energy and fundamental particles of matter.

-Matter and energy have a wave- particle duality.

- Prince Louis Victor de Broglie (pronounced de-broy) put together the ideas of Planck

and Einstein and formulated a relationship between wavelength and momentum of both waves and particles.

Significance of de Broglie's hypothesis

Bohr's Theory

 

 

Heisenberg Uncertainty Principle 

 

 

 

......................................................................................................................................